We've looked at circular and radial paths in a spherically symmetrical gravitational field, but many circumstances involve more complicated paths. For example, suppose we place highly accurate cesium clocks in an airplane and fly it around in a circle with a 100 mile radius above an airport on the equator. Assume that for the duration of the experiment the Earth has uniform translational velocity and rotates once per 24 hours. In terms of an inertial coordinate system whose origin is at the center of the Earth, the coordinates of the plane are
where R is the radius of the Earth plus the height of the airplane above the Earth's surface, W is the Earth's rotational speed, r is the radius of the circular flight path, and w is the airplane's angular speed. Differentiating these inertial coordinates with respect to the coordinate time t gives expressions for dx/dt, dy/dt, and dz/dt. Now, the proper time of the clock is given by the integral of dt over its worldline. Neglecting (for the moment) the effect of the Earth's gravitational field, we have
so we can divide through by (dt)2 and take the square root to give
Therefore, if we let V and v denote the speeds RW and rw respectively, the elapsed proper time for the clock corresponding to T of inertial coordinate time is given exactly by the integral
Since all the dimensionless parameters V, v, rW are extremely small compared to 1, we can approximate the square root very closely using 
1 - u/2, which gives the easily integrable expression
Subtracting the result from T gives the amount of dilation for the path in question. The result is
Only the first on the right is multiplied by T, so it represents the secular contributions to the time dilation, i.e., the parts that grow in proportion to the total elapsed time, whereas the two right hand terms are cyclical and don't accumulate as T increases. Not surprisingly, if we set v = r = 0 the amount of dilation is simply V2 T / 2, which is the dilation for the fixed point at the airplane's height above the equator, due entirely to the Earth's rotation. On the other hand, if we take the following values
we find that the clock fixed at a point on the equator runs slow by 100.69 nsec per 24 hours relative to our Earth-centered inertial coordinate system, whereas a clock going around in a circle of radius 100 miles at 600 mph would lose 134.99 nsec per 24 hours (neglecting the cyclical components).
Another experiment that could be performed is to fly clocks completely around the Earth's equator in opposite directions, so the eastbound clock's flight speed (relative to the ground) would be added to the circumferential speed of the Earth's surface due to the Earth's rotation, whereas the westbound clock's flight speed would be subtracted. In this situation the spatial coordinates of the clocks in the equatorial plane would be given by
where we take the + sign for the eastbound plane and - for the westbound plane. This gives the derivatives
Substituting into equation (1) and simplifying gives
Multiplying through by dt and integrating from t = 0 to some arbitrary coordinate time Dt, we find that the corresponding lapse of proper time for the plane is
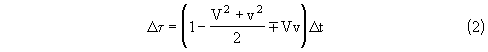
It follows that the lapse of time on the westbound clock by any coordinate time Dt will exceed the lapse of time on the eastbound clock by 2(Dt)Vv.
To this point we have neglected the gravitational field of the Earth by assuming that the metric of spacetime was the flat Minkowski metric. To account for the effects of gravity we should really use the Schwarzschild metric (assuming a spherical Earth). We saw in Section 6.4 that the metric in the equatorial plane of a spherical gravitating body of mass m at a constant Schwarzschild radial parameter r from the center of that body is
where t is the proper time along the path, t is the coordinate time, and f is the longitude. Dividing through by (dt)2 and taking the square root of both sides gives
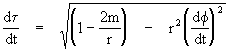
Let R denote the "radius" of the Earth, and let r = R + h denote the radius of the airplane's flight path at the constant altitude h. If we again let V denote the tangential speed of the Earth's rotation at the airplane's radial position at the equator, and let v denote the tangential speed of the airplane (either eastward or westward), we have df/dt = (V v)/r, so the above equation leads to the integral for the elapsed proper time along a path in the equatorial plane at radial parameter r = R+h from the Earth's center and with a tangential speed (relative to a fixed position above a point on the Earth's surface) is
Again making use of the approximation 1 - u/2 for small u, we can integrate this over some interval Dt of coordinate time to give the corresponding lapse Dt of proper time along the path
Naturally this is the same as equation (2) except for the extra term -2m/r, which represents the effect of the gravitational field. The mass of the Earth in gravitational units is about m = 0.0044 meters = (1.4766)10-11 sec, and if the airplanes are flying at an altitude of h = 6 miles above the Earth's surface we have r = 3986 miles = 0.021031 sec. Also, assume the speed of the airplanes (relative to the ground) is v = 500 mph, which is v = (0.747)10-6 in dimensionless units, compared with the tangential speed of the Earth's surface at the equator V = (1.527)10-6. In these conditions the above formula gives the relation between coordinate time and elapsed proper time for a clock sitting stationary at the equator on the Earth's surface as
whereas for clocks flying at an altitude of 6 miles and 500 mph eastward and westward the relations are
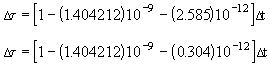
This shows that the difference in radial location between the clock on the Earth's surface and the clock up at flight altitude results in a slowing of the Earthbound clock's proper time relative to the airplane clocks of about (2.073)10-12 seconds per second of coordinate time. On the other hand, the eastbound clock has a relative slowing (compared to the Earthbound clock) in the amount of (1.419)10-12 seconds per second due to is greater speed, so the net effect is that the eastbound clock's proper time runs ahead of the Earthbound clock by about (0.654)10-12 seconds per second of coordinate time. In contrast, the westbound clock is actually moving slower than the Earthbound clock (because it's flight speed counteracts the rotation of the Earth), so it gains an additional (0.862)10-12 seconds per second. The net effect is that the westbound clock's proper time runs ahead of the Earthbound clock by a total of (2.935)10-12 seconds per second of coordinate time.
These effects are extremely small, but if an experiment is performed for an extended period of time the differences in elapsed time on highly accurate cesium clocks is large enough to be detectable. Since there are 86400 seconds in a day, we would expect to see the eastbound and westbound flying clocks in advance of the Earthbound clock by 57 nanoseconds and 254 nanoseconds respectively. Experiments of this type have actually been performed, and the results have agreed with the predictions of relativity. Notice that the "moving" clocks actually show greater lapses of proper time than the "stationary" clock, seeming to contradict special relativity, but the explanation (as we've seen) is that the gravitational effects of general relativity override the velocity effects in these particular circumstances.
Suppose we return to our original problem, which involved airplanes flying in a small circle around a fixed point on the Earth's equator, but now we want to include the effects of the Earth's gravity. The principles are the same as in the circumnavigating case, i.e., we need only integrate the proper time along the path, making use of the Schwarzschild metric to give the correct line element. However, the path of the airplane in this case is not so easy to express in terms of the usual Schwarzschild polar coordinates. One way of approaching a problem such as this is to work with the Schwarzschild metric expressed in terms of "orthogonal" quasi-Minkowskian coordinates. If we split up the coefficient of (dr)2 into the form 1 + 2m/(r-2m), then the usual Schwarzschild metric can be written as
Now if we define the quasi-Euclidean parameters
we recognize the last three terms of the preceding equation as just the expression of
(dx)2 + (dy)2 + (dz)2 in polar coordinates. Also, since r = 
we have
dr = (x dx + y dy + z dz) / r, so the Schwarzschild metric can be written in the quasi-Minkowskian form
This form is similar to Riemann normal coordinates if we expand this metric about any radius r. Also, for sufficiently large r the quantity 2m in the denominator of the final term becomes negligible, and the coefficient approaches -2m/r3, so it isn't surprising that this is one of the characteristic magnitudes of the sectional curvature of Schwarzschild spacetime at radius r. Expanding the above expression, we find that the Schwarzschild metric can be expressed as a sum of the Minkowski metric plus some small quantities as shown below
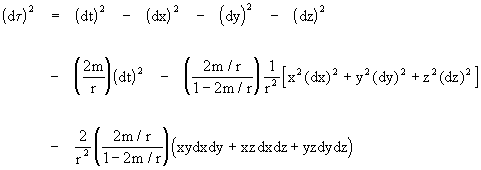
Thus in matrix notation the Schwarzschild metric tensor for these coordinates is
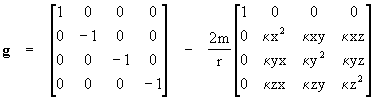
where k = 1 / [r2(1 - 2m/r)]. The determinant of this metric is -1. Dividing the preceding expression by (dt)2 and taking the square root of both sides, we arrive at a relation between dt and dt into which we can substitute the expressions for x,y,z, r, dx/dt, dy/dt, and dz/dt, and then integrate to give the proper time Dt along the path as a function of coordinate time Dt. Hence if we know x,y, and z as explicit functions of t along a particular path, we can immediately write down the explicit integral for the lapse of proper time along that path.