4.3 Free-Fall Equations

The equation of two-body non-rotating radial free-fall in Newtonian theory is formally identical to the one-body radial free-fall solution in Einstein's theory (as is Kepler's third law), provided we identify Newton's radial distance with the Schwarzschild parameter r, and Newton's time with the proper time of the falling particle. Therefore, it's worthwhile to explicitly derive the cycloidal form of this solution. From the Newtonian point of view we can begin with the inverse-square law of gravitation for the radial separation s(t) between two identical non-rotating particles of mass m

where dots signify derivatives with respect to time. Integrating this over ds from an arbitrary initial separation s(0) to the separation s(t) at some other time t gives

Notice that the left hand integral can be rewritten

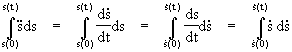
Therefore, the previous equation can easily be integrated to give

which shows that the quantity

is invariant for all t. Solving the equation for , we have

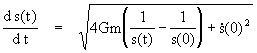
Rearranging, this gives

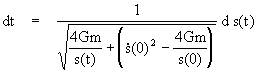
To simplify the expressions, we put s₀ = s(0), v₀ = and r = s(t)/s₀. In these terms, the preceding expression can be written

where

There are two cases to consider. If K is positive, then the trajectory is bounded, and there is some point on the trajectory (the apogee) at which v = 0. Choosing this point as our time origin t = 0, we have K=1, and the standard integral gives

(1)

This equation describes a (scaled) cycloidal relation between t and r, which can be expressed parametrically in terms of a fictitious angle q as follows

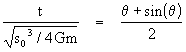
To verify that these two equations are equivalent to the preceding equation, we can solve the second for q and substitute into the first to give

(2)

Using the trigonometric identity we see that the first term on the right side is

Also, letting f = invcos(2r-1), we can use the trigonometric identity

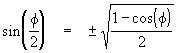
to show that this angle is

so the second term on the right side of (2) is

which completes the demonstration that the cycloid relation given by (2) is equivalent to the free-fall relation (1).

The second case is when K is negative. For this case we can conveniently express the equations in terms of the positive parameter k = -K. The standard integral

tells us that, for any two points s₀ and s₁ on the trajectory, the time interval is related to the separations according to

where

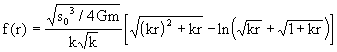
Notice that if we define S₀ = s₀ / k and R = k r, then this becomes

Thus, if we define the normalized time parameter

then the normalized equation of motion is

(3)

This represents the shape of every non-rotating separation between identical particles of mass m for which k is positive, which means that the absolute value of v₀ exceeds 2. These are the unbound radial orbits for which R goes to infinity, as opposed to the case when the absolute value of v₀ is less than this threshold, which gives bound radial orbits in the shape of a cycloid in accord with equation (1).

It's interesting to note the "removable singularity" of (3) at R = 0. Physically the parameter R is always non-negative by definition, so it abruptly reverses slope at the origin, even though the position may vary monotonically with respect to an external coordinate system.