2.6 Mobius Transformations and The Night Sky

Any proper orthochronous Lorentz transformation (including ordinary rotations and relativistic boosts) can be represented by

X ® QXQ*(1)

where

and Q* is the transposed conjugate of Q. The coefficients a,b,c,d of Q are allowed to be complex numbers, normalized so that ad - bc = 1. Just to be explicit, this implies that if we define

a = a₁ + i a₂b = b₁ + i b₂c = c₁ + i c₂d = d₁ + i d₂

then the Lorentz transformation (1) is

t' = [ a₁b₁ + a₂b₂ + c₁d₁ + c₂d₂ ] x + [ a₁b₂ - a₂b₁ + c₁d₂ - c₂d₁ ] y

+ (1/2)[ |a|² - |b|² + |c|² - |d|² ] z + (1/2)[ |a|² + |b|² + |c|² + |d|² ] t

x' = ( a₁d₁ + a₂d₂ + b₁c₁ + b₂c₂ ) x + ( a₁d₂ - a₂d₁ - b₁c₂ + b₂c₁ ) y

+ ( a₁c₁ + a₂c₂ - b₁d₁ - b₂d₂ ) z + ( a₁c₁ + a₂c₂ + b₁d₁ + b₂d₂ ) t

y' = (- a₁d₂ + a₂d₁ - b₁c₂ + b₂c₁) x + ( a₁d₁ + a₂d₂ - b₁c₁ - b₂c₂ ) y

+ (- a₁c₂ + a₂c₁ + b₁d₂ - b₂d₁ ) z + (- a₁c₂ + a₂c₁ - b₁d₂ + b₂d₁ ) t

z' = ( a₁b₁ + a₂b₂ - c₁d₁ - c₂d₂) x + ( a₁b₂ - a₂b₁ - c₁d₂ + c₂d₁ ) y

+ (1/2)( |a|² - |b|² - |c|² + |d|²) z + (1/2)( |a|² + |b|² - |c|² - |d|² ) t

Two observers at the same point in spacetime but with different orientations and velocities will "see" incoming light rays arriving from different relative directions with respect to their own frames of reference. This is partly due to ordinary rotation, and partly due to the aberration effect described in the previous section.

Anyway, we noted above that the effect of (relativistic) aberration seems to be representable by a linear fractional transformation (LFT), and furthermore we know that ordinary rotations are also represented by LFTs via stereographic projection onto the Riemann sphere. This leads to the remarkable fact that the combined effect of any proper orthochronous (and homogeneous) Lorentz transformation on the incidence angles of light rays at a point corresponds precisely to the effect of a particular LFT on the Riemann sphere via ordinary stereographic projection from the extended complex plane. The latter is illustrated below:

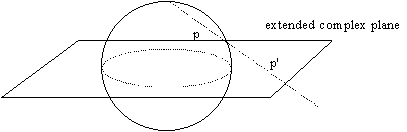
The complex number p in the extended complex plane is identified with the point p' on the unit sphere that is struck by a line from the "North Pole" through p. In this way we can identify each complex number uniquely with a point on the sphere, and vice versa. (The North Pole is identified with the "point at infinity" of the extended complex plane, for completeness.)

Relative to an observer located at the center of the Riemann sphere, each point of the sphere lies in a certain direction, and these directions can be identified with the directions of incoming light rays at a point in spacetime. If we apply a Lorentz transformation of the form (1) to this observer, specified by the four complex coefficients a,b,c,d, the resulting change in the directions of the incoming rays of light is given exactly by applying the LFT (also known as a Mobius transformation)

a,b,c,d in C, ad- bc=1 (2)

to the points of the extended complex plane. Of course, our normalization ad - bc = 1 implies the two conditions

a₁d₁ - a₂d₂ - b₁c₁ + b₂c₂ = 1

a₁d₂ + a₂d₁ - b₁c₂ - b₂c₁ = 0

so of the eight coefficients needed to specify the four complex numbers a,b,c,d, these two constraints reduce the degrees of freedom to six, which is precisely the number of degrees of freedom of Lorentz transformations (namely, three velocity components vx,vy,vz, and three angular specifications for the longitude and latitude of our line of sight, and orientation about that line of sight).

To illustrate this correspondence, first consider the "identity" Mobius transformation w ® w. In this case we have

a₁ = d₁ = 1a₂ = b₁ = b₂ = c₁ = c₂ = d₂ = 0

so our Lorentz transformation reduces to

t' = tx' = xy' = yz' = z

as expected. None of the points move on the complex plane, so none move on the Riemann sphere under stereographic projection, and nothing changes in the sky's appearance.

Now let's consider the Mobius transformation w ® - 1/w. In this case we have

b₁ = - 1c₁ = 1a₁ = a₂ = b₂ = c₂ = d₁ = d₂ = 0

and so the corresponding Lorentz transformation is

t' = tx' = - xy' = yz' = -z

This is certainly a proper orthochronous Lorentz transformation, because the determinant is +1 and the coefficient of t is positive. The question is whether reflecting the x and z coordinates agrees with the stereographic effect on the Riemann sphere of the transformation w ® - 1/w. For example, the point w = r + 0i maps to - 1/r + 0i. There's a nice little geometric demonstration that the stereographic projections of these points have coordinates (x,0,z) and (- x,0,- z) respectively, noting that the two projection lines have negative inverse slopes and so are perpendicular in the xz plane, which implies that they must strike the sphere on a common diameter (by Pythagoras' theorem). A similar analysis shows that points off the real axis with projected coordinates (x,y,z) in general map to points with projections (- x,y,- z) points.

The two examples just covered were both trivial in the sense that they left t unchanged. For a more interesting example, consider the Mobius transformation w ® w + p, which corresponds to the Lorentz transformation

t' = (1 + p²/2) t + p x - (p²/2) z

x' = pt + x - pz

y' = y

z' = (p²/2) t + p x + (1 - p²/2) z

If we denote our spacetime coordinates by the column vector X with components x₀ = t, x₁ = x, x₂ = y, x₃ = z, then the transformation can be written as

X' = LX

where

To analyze this transformation it's worthwhile to note that we can decompose any Lorentz transformation into the product of a simple boost and a simple rotation. For a given relative velocity with magnitude |v| and components v₁, v₂, v₃, let g denote the "boost factor"

It's clear that

L₀₀ = g L₁₀ = g v₁ L₂₀ = g v₂ L₃₀ = g v₃

Thus, these four components of L are fixed purely by the boost. The remaining components depend on the rotational part of the transformation. If we define a "pure boost" as a Lorentz transformation such that the two frames see each other moving with velocities (v₁,v₂,v₃) and (- v₁,- v₂,- v₃) respectively, then there is a unique pure boost for any given relative velocity vector v₁,v₂,v₃. This boost has the components

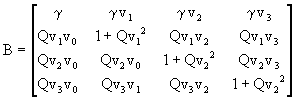
where Q = (g - 1)/|v|². From our expression for L we can identify the components to give the boost velocity in terms of the Mobius parameter p

v₂ = 0

and

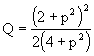
From these we write the pure boost part of L as follows

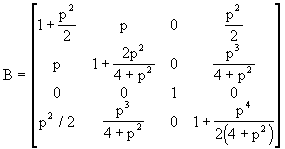
We know that our Lorentz transformation L can be written as the product of this pure boost B times a pure rotation R, i.e., L = BR, so we can determine the rotation

R = B^-1 L

which in this case gives

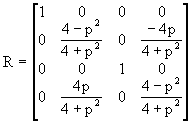
In terms of Euler angles, this represents a rotation about the y axis through an angle of

The correspondence between the coefficients of the LFT and the Lorentz transformation described above assumes stereographic projection from the North pole to the equatorial plane. More generally, if we're projecting from the North Pole of the Riemann sphere to a complex plane parallel to (but not necessarily on) the equator, and if the North Pole is at a height h above the plane, then every point in the plane is a factor of h further away from the origin than in the case of equatorial projection (h=1), so the LFT corresponding to the above Lorentz transformation is w ® (Aw+B)/(Cw+D) where

It's also worth noting that the instantaneous aberration observed by an accelerating observer does not differ from that observed by a momentarily co-moving inertial observer. We're referring here to the null (light-like) rays incident on a point of zero extent, so this is not like a finite spinning body whose outer edges have significant velocities relative to their centers. We're just referring to different coordinate systems whose origins coincide at a given point in spacetime, and describing how the light rays pass through that point in terms of the different coordinate systems at that instant. In this context the acceleration (or spinning) of the systems make no difference to the answer. In other words, as long as our inertial coordinate system has the same velocity and orientation as the (ideal point-like) observer at the moment of the observation, it doesn't matter if the observer is in the process of changing his orientation or velocity. (This is a corollary of the "clock hypothesis" of special relativity, which asserts that a traveler's time dilation at a given instant depends only on his velocity and not his acceleration at that instant.)

In general we can classify Mobius transformations (and the corresponding Lorentz transformations) according to their "squared trace", i.e., the quantity

This is also the "conjugacy parameter", i.e., two linear fractional transformations are conjugate if and only if they have the same value of s. The different kinds of transformations are listed below:

0 � s < 4elliptic

s = 4parabolic

s > 4hyperbolic

s < 0 or not realloxodromic

For example, the class of pure rotations are a special case of elliptic transformations, having the form

with

where an overbar denotes complex conjugation. Also, it's not hard to show that the compositions of an arbitrary linear fractional transformation f(z) are cyclical with a period m if and only if s = 4cos(2kp/m)².

We've seen that the general finite transformation of the incoming null rays can be expressed naturally in the form of a finite Mobius transformation of the complex plane (under sterographic projection). This is a very simple algebraic operation, given by the function

(3)

for complex constants a,b,c,d. This generates the discrete sequence f₁(z) = f(z), f₂(z) = f(f(z)), f₃(z) = f(f(f(z))), and so on for all f_n(z) where n is a positive integer. It's also possible to parameterize a Mobius transformation to give the corresponding infinitesimal generator, which can be applied to give "fractional iterations" such as f_1/2(z), or more generally the continuously parameterized transformation f_p(z) for any real (or even complex) value of p. To accomplish this we must (in general) first map the discrete generator f(z) to a domain in which it has some convenient exponential form, then apply the pth-order transformation, and then map back to the original domain. There are several cases to consider, depending on the character of the discrete generator.

In the degenerate case when ad = bc with c � 0, the pth iterate of f(z) is simply the constant f_p(z) = a/c. On the other hand, if c = 0 and a = d � 0, then f_p(z) = z + (b/d)p. The third case is with c = 0 and a � d. The pth iterate of f(z) in this case is

Notice that the second and third cases are really linear transformations, since c = 0. The fourth case is with c � 0 and (a+d)²/(ad-bc) = 4, which leads to the following closed form expression for the pth iterate

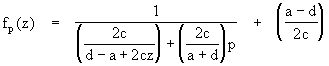
This corresponds to the case when the two fixed points of the LFT are co-incident. In this "parabolic" case, if a+d = 0 then the LFT reduces to the first case with ad-bc = 0.

Finally, in the most general case we have c � 0 and (a+d)² /(ad-bc) � 4, and the pth iterate of f(z) is given by

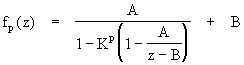
where

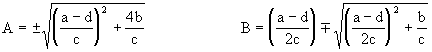
This is the general case with two distinct fixed points. (If a+d = 0 then s = 0 and K = -1.)

The parameters A and B are the coefficients of the linear transformation that maps real line to the locus of points with real part equal to 1/2. Notice that the pth composition of f satisfies the relation

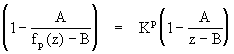
so we have

where

Thus , which shows that f(z) is conjugate to the simple function Kz. Since A+B is the complex conjugate of B, we see that h(z) can be expressed as

where

This enables us to express the pth composition of any linear fractional transformation with two fixed points, and therefore any corresponding Lorentz transformation, in the form

(4)

This shows that there is a particular oriented frame of reference, represented by h(z), with respect to which the relation between the oriented frames z and f(z) is purely exponential. (We must refer to oriented frames rather than merely frames because the Mobius transformation represented the effects of general orientation as well as velocity boost.)

To show explicitly how the action of fp(z) on the complex plane varies with p, consider the relatively simple linear fractional transformation f(z) with fixed points at 0 and 1 on the real axis, which implies A = 1 and B = 0. In parameterized form the pth composition of this transformation is of the form

for some complex constant K, and the similarity parameter for this transformation is

s = (1+K)²/K. For any given K and complex initial value z = x + iy, let

Then the real and imaginary components of f_p(z) are given by

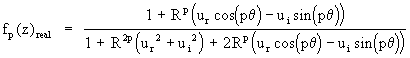
This makes explicit how the action of f_p(z) on the complex plane is entirely determined by the magnitude and phase angle of the constant K, which, as we saw previously, is given by

If a,b,c,d are all real, then s is real, in which case either K is real (s>4 or s<0) or K is complex (0<s<4) with a magnitude (norm) of 1. However, if a,b,c,d are allowed to be complex, then K can be complex with a magnitude other than 1. Of course, if K is real, we can set R = K and q = 0, so pq = 0 for all p, and the above equations reduce to

Clearly the computational complexity of the continuous parameterized transformation (4) exceeds that of the discrete transformation (3). This raises an interesting question, at least from a neo-Platonic perspective. Is it possible that nature prefers the simplicity of the discrete form over the continuous? In other words, are all physically realizable Lorentz transformations actually discrete? If so, what determines the "size" of the minimum transformation? What is the "size" of a Mobius transformation?

We know that every Mobius is conjugate to a pure exponential, and the effect of which is a rotation and a re-scaling. In addition, the conjugation itself may impose some kind of size. It's interesting that the elements now are not frames but differences between frames, including rotations. Thus, rather than the ontological objects of consideration being events of the form x,y,z,t, or even coordinate systems, the objects are the transformations with complex coefficients a,b,c,d. This again introduces the octonion space, though restricted by the fact that there are only three (complex) degrees of freedom.